Chapter 8

Question 1

A - Calculate the momentum of a $2000kg$$2000\ kg$ elephant charging a hunter at a speed of $7.50m/s$$7.50\ m/s$

B - Compare the elephant’s momentum with the momentum of a $0.040kg$$0.040\ kg$ tranquilizer dart fired at a speed of $600m/s$$600\ m/s$

• Calculate elephant's momentum

• Comparison = Ratio = Division

C - What is the momentum of the 90.0\ kg hunter running at $7.40m/s$$7.40\ m/s$ after missing the elephant?

Question 3

A - At what speed would a $2.00\ast {10}^{4}kg$$2.00 * 10^4\ kg$ airplane have to fly at to have a momentum of $1.60\ast {10}^{9}kg\cdot m/s$$1.60 * 10^9\ kg\ \cdot m/s$ ( the same as the ship's momentum in the problem above ) ?

B - What is the plane’s momentum when it is taking off at a speed of $60.0m/s$$60.0\ m/s$

C - If the ship is an aircraft carrier that launches these airplanes with a catapult, discuss the implications of your answer to (b) as it relates to recoil effects of the catapult on the ship.

• Airplanes Momentum = $1.2\ast {10}^{6}kg\cdot m/s$$1.2 * 10^6\ kg \cdot m/s$

• Ships Momentum = $1.60\ast {10}^{9}kg\cdot m/s$$1.60 * 10^9\ kg\ \cdot m/s$

• The ships momentum is 3 powers of 10 larger than the airplanes ( ${10}^9$$10^9$ vs ${10}^6$$10^6$ )

• Equation 8.30

  • Conservation of Momentum $\left(0\right)$$\left(\ 0 \ \right)$ = sum of all momentums in the system

• If we apply this to the principle of recoil :

  • the momentum forces now are going in opposite directions

  • so lets say airplane = positive direction

  • and catapult / ship = negative direction

• Ok , but instead of momentum directly , we break it down to mass times velocity again.

• We can just rearrange that equation to let us solve for the "recoil" velocity $\left(v_2\right)$$\left(\ v_2 \ \right)$ during the catapulting process

• First though , we have to go back to question 2 , and get the mass off the ship

• Finally we can plug everything in to the "recoil" equation :

• They say this recoil velocity is very small and not noticeable if you were standing on the ship

Question 15 - A cruise ship with a mass of $1.00\ast {10}^{7}kg$$1.00 * 10^7\ kg$ strikes a pier at a speed of $0.750m/s$$0.750\ m/s$. It comes to rest $6.00m$$6.00\ m$ later, damaging the ship, the pier, and the tugboat captain’s finances. Calculate the average force exerted on the pier using the concept of impulse. ( Hint: First calculate the time it took to bring the ship to rest )

• Time it took to bring the ship to rest :

• X-Axis Displacement = $\mathrm{\Delta }x$$\Delta x$

• Average Velocity = $\overline{v}$$\bar{v}$

• Time Interval = $\mathrm{\Delta }t$$\Delta t$

• Impulse, or change in momentum, equals the average net external force multiplied by the time this force acts:

• Calculate Initial Momentum :

• Calculate net force

• The pier was at rest , so it experiences this as $+4.6875\ast {10}^{5}Newtons$$+4.6875 * 10^5\ Newtons$

Question 23 - Train cars are coupled together by being bumped into one another. Suppose two loaded train cars are moving toward one another, the first having a mass of $150,000kg$$150,000\ kg$ and a velocity of $0.300m/s$$0.300\ m/s$ , and the second having a mass of $110,000kg$$110,000\ kg$ and a velocity of $-0.120m/s$$-0.120\ m/s$. ( The minus indicates direction of motion ) What is their final velocity?

• Equation 8.53

• We modify this though to just a single final velocity of the cars together :

• Algebra

• Rearrange to solve for final velocity $\left(v^{\prime }\right)$$\left(\ v^{\prime} \ \right)$ :

• $m_1$$m_1$ = mass of first train car = $1.5\ast {10}^{5}kg$$1.5 * 10^5\ kg$

• $m_2$$m_2$ = mass of second train car = $1.1\ast {10}^{5}kg$$1.1 * 10^5\ kg$

• $v_1$$v_1$ = velocity of first train car BEFORE collision = $3\ast {10}^{-1}m/s$$3 * 10^{-1}\ m/s$

• $v_2$$v_2$ = velocity of second train car BEFORE collision = $-1.2\ast {10}^{-1}m/s$$-1.2 * 10^{-1}\ m/s$

• $v^{\prime }$$v^{\prime}$ = final velocity AFTER collision = UNKNOWN

Question 25 - Consider the following question: A car moving at $10m/s$$10\ m/s$ crashes into a tree and stops in $0.26s$$0.26\ s$. Calculate the force the seatbelt exerts on a passenger in the car to bring him to a halt. The mass of the passenger is $70\ kg$. Would the answer to this question be different if the car with the $70\ kg$ passenger had collided with a car that has a mass equal to and is traveling in the opposite direction and at the same speed? Explain your answer.

• This is the book's answer to the conceptual part :

  • In a collision with an identical car, momentum is conserved.

  • Afterwards $v_f=0$$v_f = 0$ for both cars.

  • The change in momentum will be the same as in the crash with the tree.

  • However, the force on the body is not determined since the time is not known.

  • A padded stop will reduce injurious force on body.

Question 31 - A $0.240kg$$0.240\ kg$ billiard ball that is moving at $3.00m/s$$3.00\ m/s$ strikes the bumper of a pool table and bounces straight back at $2.40m/s$$2.40\ m/s$ (80% of its original speed). The collision lasts $0.0150s$$0.0150\ s$.

A - Calculate the average force exerted on the ball by the bumper.

B - How much kinetic energy in joules is lost during the collision?

C - What percent of the original energy is left?

Question 44

A - During an ice skating performance, an initially motionless $80.0kg$$80.0\ kg$ clown throws a fake barbell away. The clown’s ice skates allow her to recoil frictionlessly. If the clown recoils with a velocity of $0.500m/s$$0.500\ m/s$ and the barbell is thrown with a velocity of $10.0m/s$$10.0\ m/s$ , what is the mass of the barbell?

B - How much kinetic energy is gained by this maneuver?

• Calculate $KE$$KE$ final :

• Calculate $KE$$KE$ initial :

• Finally ,

C - Where does the kinetic energy come from?

• Book Answer :

  • The clown does work to throw the barbell ,

    • so the kinetic energy comes from the muscles of the clown.

      • The muscles convert the chemical potential energy of ATP into kinetic energy.

Question 45 - Two identical pucks collide on an air hockey table. One puck was originally at rest.

A - If the incoming puck has a speed of $6.0m/s$$6.0\ m/s$ and scatters to an angle of ${30.0}^{\circ }$$30.0^{\circ}$, what is the velocity ( magnitude and direction ) of the second puck? ( You may use the result that ${\theta }_1-{\theta }_2={90.0}^{\circ }$$\theta_1 - \theta_2 = 90.0^{\circ}$ for elastic collisions of objects that have identical masses. )

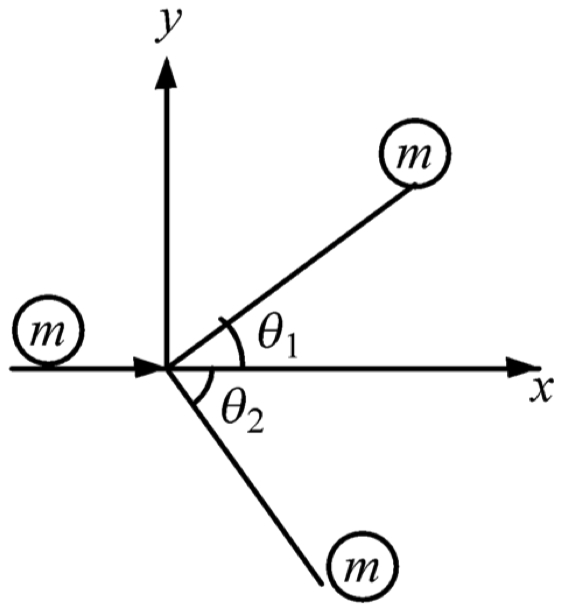
• Let ${\theta }_1={30}^{\circ }$$\theta_1 = 30^{\circ}$

• Therefore , $30-{\theta }_2=90$$30 - \theta_2 = 90$

  • $-{\theta }_2=60$$-\theta_2 = 60$

  • ${\theta }_2=-{60}^{\circ }$$\theta_2 = -60^{\circ}$

• Conservation of Momentum in X-Direction :

  • Equation 8.62

  $$m_1\ast {\nu }_1=(m_1\ast {\nu }_1^{\prime }\ast cos({\theta }_1))+(m_2\ast {\nu }_2^{\prime }\ast cos({\theta }_2))$$

• Conservation of Momentum in Y-Direction :

  • Equation 8.67

  $$0=(m_1\ast v_1^{\prime }\ast sin({\theta }_1))+(m_2\ast v_2^{\prime }\ast sin({\theta }_2))$$

• Combining the two equations :

  • First solve Equation 8.67 for $v_1^{\prime }$$v^{\prime}_1$ :

  $$(-1)\ast m_1\ast v_1^{\prime }\ast sin({\theta }_1)=m_2\ast v_2^{\prime }\ast sin({\theta }_2)$$
  $$v_1^{\prime }=\frac{m_2\ast v_2^{\prime }\ast sin({\theta }_2)}{(-1)\ast m_1\ast sin({\theta }_1)}$$

  • We can simplify though , because both hocky pucks are identical in mass

  • So just let $m_2$$m_2$ and $m_1$$m_1$ be replaced by $m_x$$m_x$ , then it just drops / cancels from the equation

  $$v_1^{\prime }=\frac{v_2^{\prime }\ast sin({\theta }_2)}{(-1)\ast sin({\theta }_1)}$$

  • Lets make the same simplification of the masses for Equation 8.62 , and rewrite it in terms of $v_2^{\prime }$$v^{\prime}_2$ :

• Now we can use these $v_1^{\prime }$$v^{\prime}_1$ and $v_2^{\prime }$$v^{\prime}_2$ primitives to substitute back into either equation really , but lets use Equation 8.62 :

• Using Wolfram Alpha to simplify :

• Calculate final velocity of one of the pucks $v_2^{\prime }$$v^{\prime}_2$ :

• Finally solve for final velocity of the other hockey puck :

B - Confirm that the collision is elastic

• Need to check if kinetic energy is conserved during the collision

  • total kinetic energy before and after the collision has to be the same

• Calculate $K{E}_{\text{initial}}$$KE_{\text{initial}}$ :

  • they didn't give us a mass , but we can ignore it anyways because the mass doesn't change from initial to final state

• Calculate $K{E}_{\text{final}}$$KE_{\text{final}}$ :

• KE final and initial have equal values , therefore the collision is perfectly elastic.